Yoshida Award for Research Paper
Method for Calculating the Design Shear Capacity of Reinforced Concrete Beams to Ensure Continuity to the Shear-Span-to-Effective-Depth Ratio
PDF Version
Yuki NAKATA Railway Technical Research Institute	Ken WATANABE Railway Technical Research Institute	Tadatomo WATANABE Hokubu Consultant Co.,Ltd.	Yukihiro TANIMURA Railway Technical Research Institute

T he safety of reinforced concrete (RC) structures subjected to shear force is verified by confirming that shear forces do not reach the design shear capacities in the standard specifications established by the Japan Society of Civil Engineers [1]. The design shear capacities are calculated using the following equations.
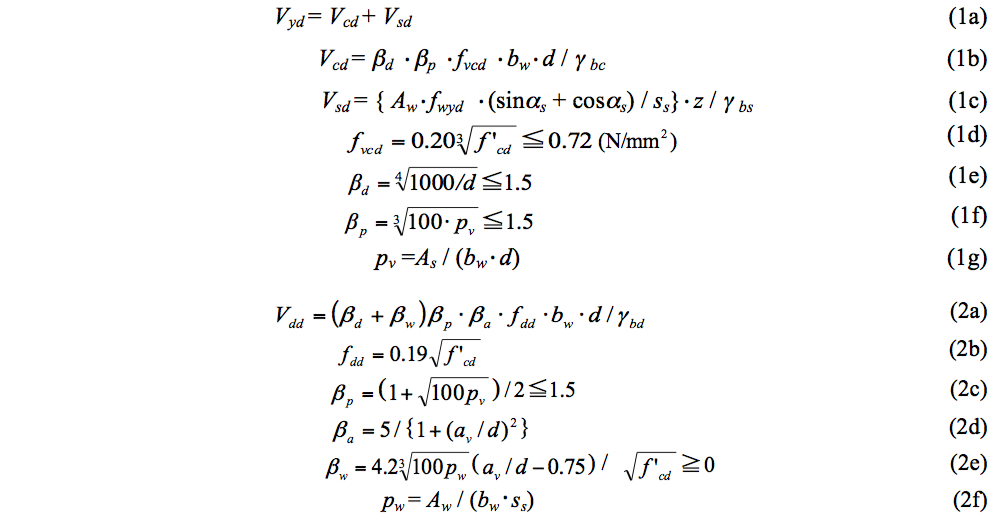When shear reinforcement ratio pw<0.002, pw is taken as 0.
The basic experimental equations behind the design equations for calculating the shear capacities are shown below.
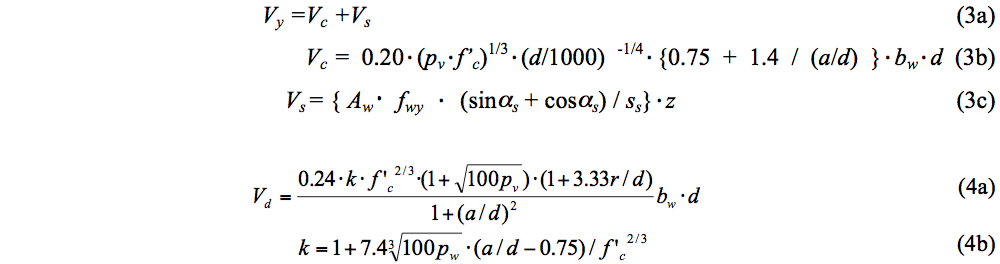When shear reinforcement ratio pw<0.002, pw is taken as 0.
The notations are explained in the standard specifications [1].
The experimental and design equations express the average values and the lower limit of the experimental results, respectively. Figures 1 and 2 show comparisons of Vyd and Vdd with experimental results Vuexp for rectangular cross-sectioned reinforced concrete beams with simple supports. Although Vyd and Vdd correspond to diagonal tension failure and shear compression failure, respectively, Vyd and Vdd express the lower limits of Vuexp. However, the experimental scopes of pw are 0 ~ 0.72% for diagonal tension failure and 0 ~ 2.58% for shear compression failure.
Figure 1: Comparison of Vyd and Vuexp	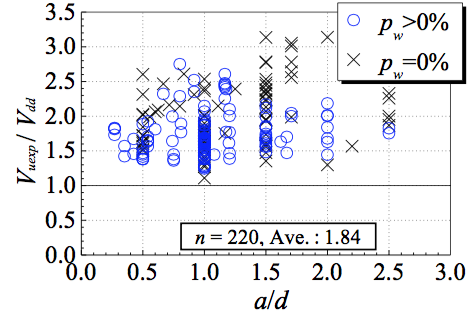Figure 2: Comparison of Vdd and Vuexp
Figure 3 shows the relationships of the shear capacities and a/d for a specified section (such as the transverse beams of railway viaducts). Though the equations are expressed as a function of the shear span to the effective depth ratio (a/d), Vyd is used in a/d ≧ 2.0 and Vdd is used in a/d < 2.0 [1]. Vyd and Vdd at a/d = 2.0 show a significant difference (Vyd /Vdd =2.5), and Vyd is larger than Vdd at any a/d. This difference means that Vyd overestimates and/or Vdd underestimates the actual shear capacities if these have the continuity to a/d. The calculations show that the significant differences between Vyd (Vy) and Vdd (Vd) at a/d = 2.0 occur when pw and/or yield strength of shear reinforcement fwyd is large.
In addition, the differences between Vyd and Vy are not the same as those between Vdd and Vd from Figure 3. The differences between the design equations and the experimental equations at the same a/d depend on the degree of reliability of the experimental equations. This is because the design equations Vyd and Vdd express the lower limits of Vuexp.
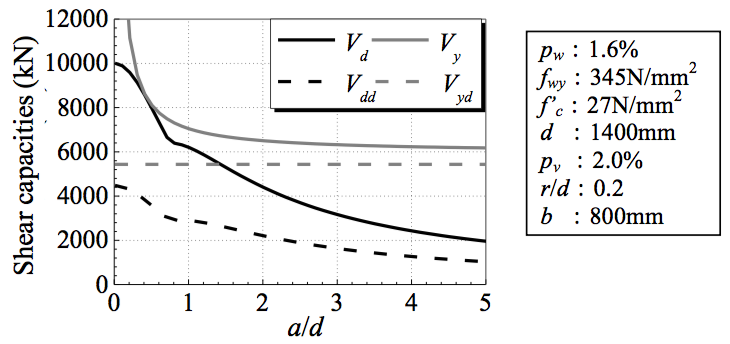Figure 3: Continuity of calculated values for shear capacity to a/d
Research to date has used nonlinear finite element analysis to estimate the shear capacities for RC beams with large shear reinforcement. This research shows that the shear capacities of RC beams with vertical shear reinforcement are properly calculated using the upper limit of Vs from equation 5.
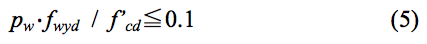Figure 4 shows comparisons of Vuexp and Vyd (=Vcd + Vsd) when equation 5 is considered. Moreover, according to research to date, the upper limit of fwyd has been 25 f’cd (f’cd : design compressive strength of concrete). It is confirmed that Vyd contains all Vuexp when equation 5 is considered.
Figure 4: Comparison of Vyd (when equation 5 is considered) and Vuexp
Next, a method for considering r (r: length of bearing plate along the longitudinal axis) for Vdd is reevaluated. This is difficult to set up when calculating the shear capacities of actual structures. Vdd of equation 2 is condensed from Vd to r/d = 0.05. Figure 5 shows a comparison of Vdd_pw, which is condensed to r/d = 0.10, and Vuexp for RC beams with shear reinforcement. A comparison of the experimental results confirmed that Vdd contains all Vuexp for RC beams with shear reinforcement, even if r/d is 0.10. Vdd_pw for RC beams with shear reinforcement is calculated using equation 6.
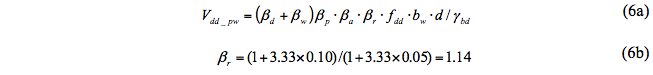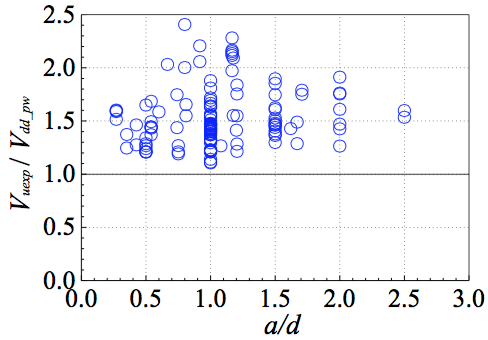Figure 5: Comparison of Vdd_pw (r/d = 0.10) and Vuexp (pw > 0 %)
From the above results, the following equation is proposed as a method for calculating the design shear capacity to ensure continuity to a/d.
Vyd is considered equation 5.
Figure 6 shows comparisons of Vdesign and Vuexp. It has been confirmed that Vdesign contain almost all of Vuexp. Figure 7 shows the relationships of the shear capacities and a/d for the same section in Figure 3. The proposed method can ensure continuity of the design shear capacity to a/d.
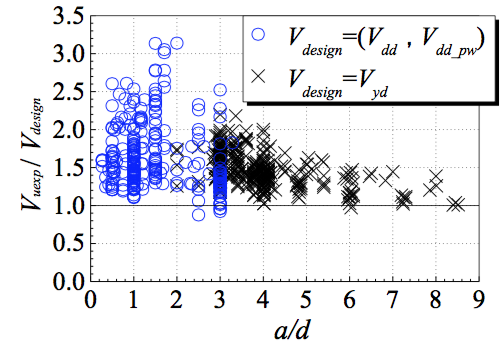Figure 6: Comparison of Vdesign and Vuexp	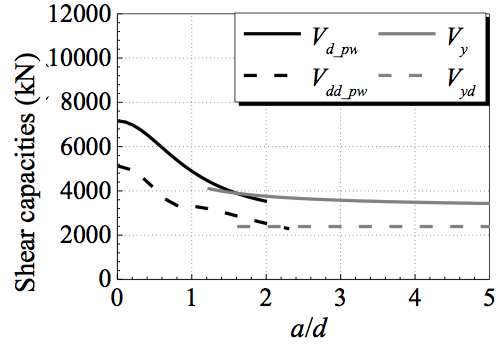Figure 7: Continuity of calculated values of shear capacities to a/d using Vdesign
Reference [1] Japan Society of Civil Engineers: Standard specifications for concrete structures - 2007 “Design,” JSCE Guidelines for Concrete No. 15, 2010.12.